JETP Letters: issues online

We investigate the thermodynamic properties of inner and outer horizons in the background of spinning BTZ (Ba 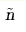$\tilde{\text{n}}$ ados, Teitelboim, and Zanelli) black hole. We compute the horizon radii product, the entropy product, the surface temperature product, the Komar energy product, and the specific heat product for both the horizons. We observe that the entropy product is universal (mass-independent), whereas the surface temperature product, Komar energy product, and specific heat product are not universal because they all depends on mass parameter. We also show that the First law of black hole thermodynamics and Smarr-Gibbs-Duhem relations hold for inner horizon as well as outer horizon. The Christodoulou-Ruffini mass formula is derived for both the horizons. We further study the stability of such black hole by computing the specific heat for both the horizons. It has been observed that under certain condition the black hole possesses second order phase transition.