Home
For authors
Submission status

Archive
Archive (English)
Current
   Volumes 113-121
   Volumes 93-112
      Volume 112
      Volume 111
      Volume 110
      Volume 109
      Volume 108
      Volume 107
      Volume 106
      Volume 105
      Volume 104
      Volume 103
      Volume 102
      Volume 101
      Volume 100
      Volume 99
      Volume 98
      Volume 97
      Volume 96
      Volume 95
      Volume 94
      Volume 93
Search
VOLUME 99 (2014) | ISSUE 1 | PAGE 45
Line bundle twisted chiral de Rham complex, chiral Riemann-Roch formula and D-branes on toric manifolds
DOI: 10.7868/S0370274X14010093
Abstract
I represent the results of the elliptic genus calculations in various examples of twisted chiral de Rham complex on one- and two-dimensional toric compact manifolds. The explicit calculations are made for line bundle twisted chiral de Rham complex on 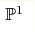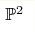\mathbb{P}^{1}, \mathbb{P}^{2} and Hirzebruch surface. Based on these results I propose the elliptic genus expression of the bundle twisted chiral de Rham complex for general smooth compact two dimensional toric manifold. The expression resembles Riemann-Roch formula and coincides with the later in certain limit. I interprete the result in terms of infinite tower of open string oscillator contributions and identify directly the open string boundary conditions of the corresponding bound state of D-branes.